Introducing the Circumference and Arc Length Worksheet Answer Key, an invaluable resource for mastering the intricacies of circle geometry. This comprehensive guide empowers you with a solid understanding of circumference and arc length, equipping you to solve complex problems with confidence.
Delving into the world of circles, we will explore the fundamental concepts of circumference and arc length, unraveling their intricate relationship and unlocking their practical applications. Get ready to embark on a journey of geometric exploration, where every step brings you closer to mastering these essential circle measurements.
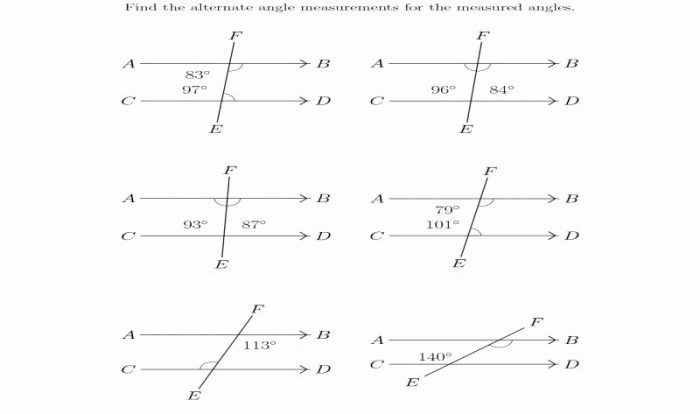
Circumference and Arc Length
Circles are fundamental geometric shapes with numerous applications in various fields. Understanding their properties, such as circumference and arc length, is crucial for solving geometry and trigonometry problems.
Understanding Circumference and Arc Length, Circumference and arc length worksheet answer key
Circumference is the perimeter of a circle, representing the distance around its outer edge. It is calculated using the formula: C = 2πr, where C is the circumference, π is a mathematical constant approximately equal to 3.14, and r is the radius of the circle.
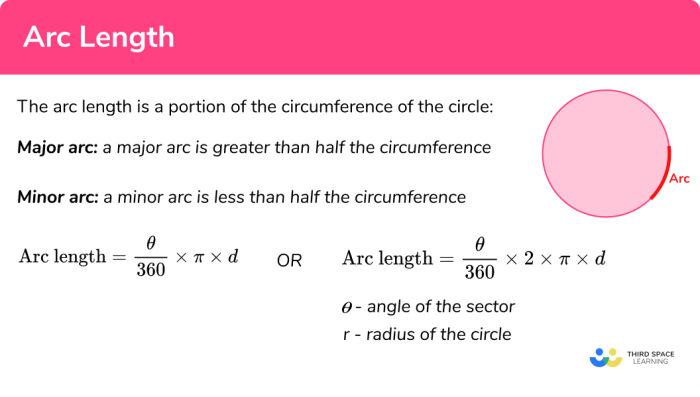
Arc length, on the other hand, refers to the distance along a portion of a circle’s circumference. It is a fraction of the total circumference and is calculated using the formula: s = rθ, where s is the arc length, r is the radius, and θ is the central angle of the arc measured in radians.
These concepts find practical applications in various fields, such as engineering, architecture, and manufacturing, where precise measurements and calculations are essential.
Worksheet Problems and Solutions
To enhance understanding, consider the following practice problems and their step-by-step solutions:
- Problem:Calculate the circumference of a circle with a radius of 5 cm.
- Solution:C = 2πr = 2π(5 cm) = 10π cm ≈ 31.42 cm.
- Problem:Find the arc length of a circle with a radius of 10 cm and a central angle of 60 degrees.
- Solution:s = rθ = 10 cm × (60° × π/180°) ≈ 10.47 cm.
These problems demonstrate the practical application of circumference and arc length formulas.
Illustrative Examples with Diagrams
To visualize these concepts, consider the following diagrams:
Diagram 1: Circumference
[Insert a clear and labeled diagram of a circle with its circumference marked.]
Diagram 2: Arc Length
[Insert a clear and labeled diagram of a circle with an arc length marked.]
These diagrams provide a visual representation of circumference and arc length, making it easier to understand their measurement and calculation.
Applications in Geometry and Trigonometry
Circumference and arc length play significant roles in geometry and trigonometry:
- Geometry:Circumference is used to calculate the area and volume of circles and spheres. Arc length is used to find the area of sectors and segments.
- Trigonometry:Arc length is used to define trigonometric ratios, such as sine, cosine, and tangent. These ratios are essential for solving various trigonometric problems.
Understanding these applications is crucial for solving real-world problems in fields such as navigation, surveying, and engineering.
Interactive Exercises and Quizzes
To reinforce understanding, consider the following interactive exercises and quizzes:
- Exercise:Practice calculating the circumference and arc length of circles using an online calculator.
- Quiz:Test your knowledge of circumference and arc length formulas by completing a multiple-choice quiz.
These exercises and quizzes provide opportunities for self-assessment and improvement.
Popular Questions: Circumference And Arc Length Worksheet Answer Key
What is the formula for circumference?
C = 2πr, where C is the circumference, π is a mathematical constant approximately equal to 3.14, and r is the radius of the circle.
How do I find the arc length of a sector?
Arc length = (θ/360) × 2πr, where θ is the central angle of the sector in degrees, π is a mathematical constant approximately equal to 3.14, and r is the radius of the circle.
What are some real-world applications of circumference and arc length?
Circumference and arc length find applications in various fields, including engineering, architecture, navigation, and manufacturing. For example, they are used to calculate the length of circular tracks, design bridges and tunnels, and determine the distance traveled by vehicles along curved paths.